Applications to the computation of the integrals of plane figures areas. Calculation the arc length, the amount of body rotation. The improper integral

LECTURE PLAN:

1. Application to the computation of definite integrals of plane figures areas

2. Calculations of the length, the amount of body rotation

3. The improper integral

Applications of definite integrals

The areas of plane figures

If a continuous curve is defined in rectangular coordinates by the equation the area of the curvilinear trapezoid bounded by this curve, by two vertical lines at the

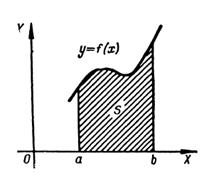
points x=a and x =b and by a segment of the x-axis , is given by the formula

.

In the more general case, if the area S is bounded by two continuous curves and and by two vertical lines x=a and x=b, where 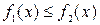when , we will then have:

.

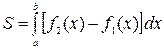If the curve is defined by equations in parametric form 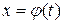and then the area of the curvilinear trapezoid bounded by this curve, by two vertical lines (x=a and x=b), and by a segment of the x-axis is expressed by the integral

,

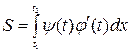where 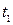and 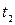are determined from the equations and 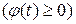on the interval .

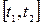If a curve is defined in polar coordinates by the equation 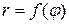, then the area of the sector AOB (Fig. 2), bounded by an arc of the curve, and by two radius vectors OA and OB,

Fig. 2.

which correspond to the values and is expressed by the integral

.

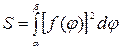The arc length of a curve

The arc length s of a curve y=f(x) contained between two points with abscissas x=a and x=b is

.

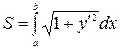If a curve is represented by equations in parametric form 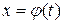and then the arc length s of the curve is

,

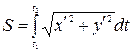where 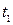and 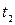are values of the parameter that correspond to the extremities of the arc.

If a curve is defined by the equation 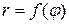in polar coordinates, then the arc length s is

,

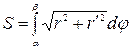where 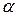and are the values of the polar angle at the extreme points of the arc.

The volume of a solid of revolution

The volumes of solids formed by the revolution of a curvilinear trapezoid [bounded by the curve y=f(x), the x-axis and two vertical lines x=a and x=b] about the x-axis and y-axes are expressed, respectively, by the formulas:

and .

The Area of a Surface of Revolution

The area of a surface formed by the rotation, about the x-axis, of an arc of the curve y=f(x) between the points x=a and x=b, is expressed by the formula

.

If a curve is represented by equations in parametric form 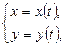then ,

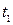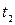where and are values of the parameter t.

Date: 2015-01-02; view: 1019