Parabola

Definition. The locus of points for which the distance to a fixed point equals the distance to a given straight line (a directrix) is called a parabola.

Let us draw the perpendicular through a fixed point to the given straight line and take it for the x-axis. From the middle point of the segment joining the focus to the given straight line we draw a perpendicular and take it for the y-axis.

p М(х;у)

r

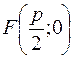0 x

To derive the equation of the parabola, we take an arbitrary point М(х;у) on it and write down the characteristic feature of a parabola as a mathematical formula.

The distance from the focus to the directrex is called the parameter of the parabola and denoted by p. Let us find the distance from the point М(х;у) to the focus:

,

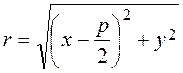and = .

By definition, these distances are equal:

.

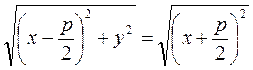Let us transform this, relation by squaring both sides:

; .

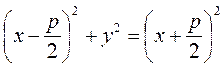We obtain

. (20)

This is the classical equation of a parabola.

The parabola passes through the origin (0;0), because it satisfies equation (20).

Suppose that the parameter is a positive number р>0; then, since у²>0, we have x>0, and the parabola is contained in the right half-plane. If p<0, then x<0, and the parabola is contained in the left half-plane

y у

p>0 p<0

0 x 0 х

M₀(x₀,y₀)

Consider the equation of a parabola in the “school” form 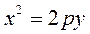. Let us analyze this equation by analogy with (20): if p>0, then y>0, and the branches of the parabola are directed upward; if p<0, then y<0, and the branches of the parabola are directed downward.

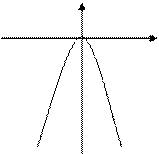
p>0 y p<0 y

0 x

0 x

The eccentricity of the parabola, that is, the ratio of the focal radius to the distance from a point to the directrix, equals 1, i.e.,

.

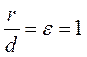Date: 2015-01-02; view: 1064