
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
Asymptotes of a Hyperbola
Definition. The asymptote of a curve is a straight line approached by the curve line at infinity.
An oblique asymptote is determined by an equation of the form an oblique
y=kx+b.
To find the slope, we suppose that b=0; then
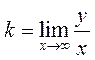 ;
; 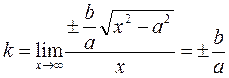 .
.
We find b from the equation 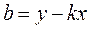 .
.
Passing to the limit, we obtain
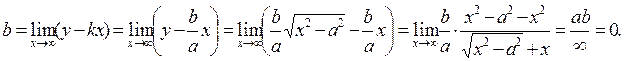
Thus, the hyperbola has two asymptotes, passing through the origin:
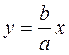 ;
; 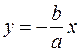 .
.
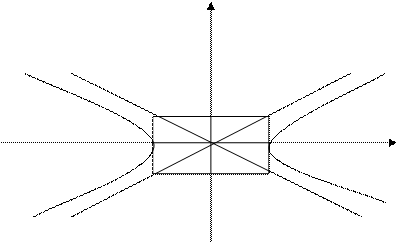
First, we construct a rectangle with sides 2à along the õ-axis and 2b along the ó-axis. We draw the diagonals in this rectangle and extend them; they are the asymptotes of the hyperbola.
 y
y 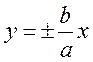
2a 2b


F1 Ì1(–à;0) 0 Ì2(à;0) F2 x
From the vertices M1(–a;0) and M2(a;0), we draw the branches of the hyperbola approaching the asymptotes.
The directrices of a hyperbola. By definition, . By analogy with an ellipse, we find the difference of squares
 ,
,
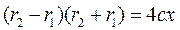 .
.
Date: 2015-01-02; view: 1488
| <== previous page | | | next page ==> |
| Tangents to an Ellipse | | | As a result, we obtain |