Turning Points

In the absence of a nonconservative force, the mechanical energy of the system has a constant value given by

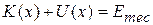. (8-21)

Here is the kinetic energy function of the particle (this gives the kinetic energy as a function of the particle's location x). We may rewrite Eq. 8-21 as

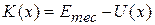(8-22)

Suppose that (which has a constant value, remember) happens to be 5.0 J. It would be represented in Fig. 8-10a by a horizontal line that runs through the value 5.0 J on the energy axis. (It is, in fact, shown there.)

Equation 8-22 tells us how to determine the kinetic energy 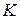for any location x of the particle: On the 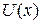curve, find U for that location x and then subtract U from For example, if the particle is at any point to the right of , then К = 1.0 J. The value of К is greatest (5.0 J) when the particle is at x₂, and least (0 J) when the particle is at x_v

Since 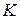can never be negative (because v² is always positive), the particle can never move to the left of _? where 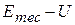is negative. Instead, as the particle moves toward from. x₂, К decreases (the particle slows) until К = 0 at (the particle stops there).

Note that when the particle reaches , the force on the particle, given by Eq. 8-20, is positive (because the slope is negative). This means that the particle does not remain at but instead begins to move to the right, opposite its earlier motion. Hence is a turning point, a place where 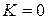(because 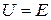) and the particle changes direction. There is no turning point (where 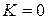) on the right side of the graph. When the particle heads to the right, it will continue indefinitely.

Equilibrium Points

Figure 8-10c shows three different values for superposed on the plot of the same potential energy function 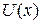. Let us see how they would change the situation. If = 4.0 J (purple line), the turning point shifts from to a point between and x₂. Also, at any point to the right of , the system's mechanical energy is equal to its potential energy; thus, the particle has no kinetic energy and (by Eq. 8-20) no force acts on it, and so it must be stationary. A particle at such a position is said to be in neutral equilibrium. (A marble placed on a horizontal tabletop is in that state.)

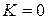If = 3.0 J (pink line), there are two turning points: One is between and , and the other is between and . In addition, is a point at which . If the particle is located exactly there, the force on it is also zero, and the particle remains stationary. However, if it is displaced even slightly in either direction, a nonzero force pushes it farther in the same direction, and the particle continues to move. A particle at such a position is said to be in unstable equilibrium. (A marble balanced on top of a bowling ball is an example.)

Next consider the particle's behavior if = 1.0 J (green line). If we place it at , it is stuck there. It cannot move left or right on its own because to do so would require a negative kinetic energy. If we push it slightly left or right, a restoring force appears that moves it back to : A particle at such a position is said to be in stable equilibrium. (A marble placed at the bottom of a hemispherical bowl is an example.) If we place the particle in the cuplike potential well centered at , it is between two turning points. It can still move somewhat, but only partway to or .

Date: 2015-01-12; view: 911