Fundamental Theorems on Limits

Theorem I. The limit of the algebraic sum of finitely many functions equals the sum of the limits of these functions:

.

Theorem II. The limit of the product of two functions equals the product of the limits of these functions:

.

Theorem III. The limit of the ratio of two functions equals the quotient of the limits of the numerator and the denominator:

.

Computations of limits. Examples.

I. Limits as x.

(1)

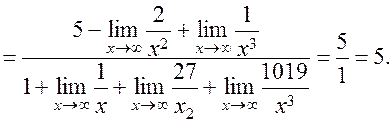
The limits in the numerator and the denominator equal zero.

To find the limit of a linear-fractional function, we must divide the numerator and the denominator by х to the maximum power among the powers of x in the numerator and the denominator.

(2)

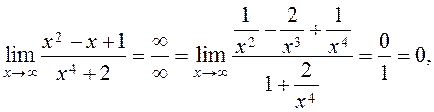
because х⁴ is the maximum power of x in the numerator and the denominator.

(3) 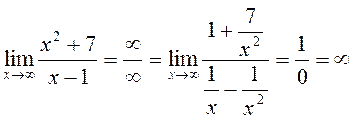(divide by х²).

A simple method for finding limits of linear-fractional functions as х is to leave the term containing the maximum power of х in the numerator and the denominator:

4) ,

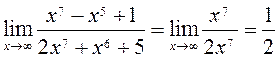5) ,

6) .

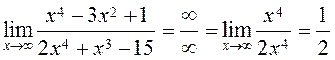Let us find limits (1), (2), (3) by the simple method:

,

,

.

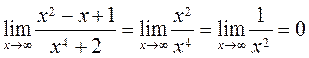Deleting the terms containing lower powers of x from the numerator and the denominator is only possible because, after division by х to the maximum power, the limits of all such terms vanish.

II. Limits as ха. Looking for a limit, first, substitute 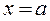in the function. If we obtain a number, then this number is the limit of the function. If we obtain one of the indeterminacies 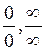,1^, and , then we must eliminate it by transforming the function and then to pass to the limit.

(1) ,

(2) ,

(3) ,

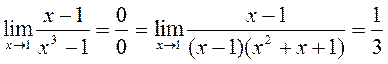The First Remarkable Limit and Its Generalization

The following limit exists and equals 1:

.

Example:

(1) ,

2) .

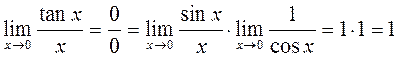The first generalized remarkable limit. The first remarkable limit can be generalized, namely, written in the more general form

(4)

In this formula, (х) is an infinitesimal; it is very important that the argument of the sine and the denominator must be absolutely identical.

Examples.

(1) ,

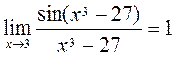(2) .

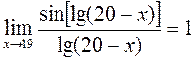The Second Remarkable Limit

Consider the limit

. (5)

The number е satisfies the inequalities 2<e<3, e is an abbreviation for exponentials, i.e., «outer»; it is sometimes denoted by e=exp and approximately equals e2,71828. y=e^x is the exponential function.

Examples. Find the following limits by using the second remarkable limit:

.

The Second Generalized Remarkable Limit

The second remarkable limit (*) and its modification (**) can be generalized, i.e., written in the more general forms

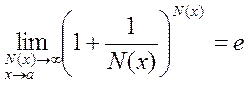
and

. (6)

In these formulas, (х) is an infinitesimal and N(x) is an infinitude. It is very important that in these formulas, N(x) and (х) are absolutely identical in the denominators and exponents.

For example,

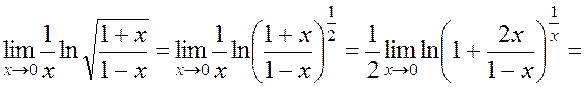
Other Remarkable Limits

Consider the following limits of functions often encountered in applications:

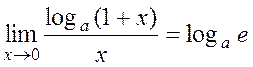, (7)

for a=e, . (8)

. (9)

Date: 2015-01-02; view: 3166