
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
INFLUENCE OF EXPECTED RATE OF RETURN ON STOCK AND BOND PRICES
What is the expected return on a share of stock? It is the expected dividend plus the expected change in the price of the stock, all divided by the share price at the time of purchase:
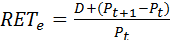 (4.2)
(4.2)
where D – expected dividend;
Pt – price at time of purchase;
Pt+1 – expected price at the end of time period.
For example, if the stock price at the time of purchase is 1000 ˆ, the expected dividend is 40 ˆ per share and the expected increase in the price over year is 40 ˆ, the expected return is 8%:
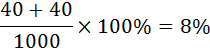
The same result can be received under the following circumstances:
| Expected dividend | Expected price change (capital gain (+) or loss (-)) | Expected return |
| (80+0)/1000 × 100% = 8% | ||
| (40+40)/1000 × 100% = 8% | ||
| (0+80)/1000 × 100% = 8% |
What is the expected return on a bond? It is the coupon rate plus the expected percentage change in the bond's price over the course of the year:
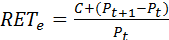 (4.3)
(4.3)
where C – coupon payment;
Pt – price at the beginning of the year;
Pt+1 – expected price at the end of the year.
For example, expected percentage return on newly issued 5-year bond with face value 1000 and coupon rate 6% in a year after interest rates fall to 4% is equal to
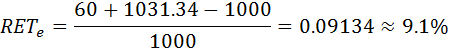

To see how bond prices are connected with stocks prices, assume that stocks and bonds have the same degree of liquidity, the current interest rate on bonds is 6 percent and that the expected return on stocks is 8 percent, and the expected capital gain is zero.
As bonds are less risk assets than stocks[3], the stockholder should be compensated additional 2 % of the return:
risk-adjusted return on stocks = risk-adjusted return on bonds (4.4)
8% (return on stocks) – 2% (risk premium) = 6% (return on bonds)
From the investor’s point of view in such case the stocks and bonds are equally attractive, and he is indifferent between stocks and bonds.
Now suppose that the Central bank decides to increase money supply within carrying out expansionary monetary policy. It makes downward pressure at interest rates which decrease from 6 to 4%, reducing, in turn, the risk-adjusted return on bonds from 6 to 4%.
The fall in the interest rate will tend to raise stock prices through two channels.
First, the expected return on bonds is now below the risk-adjusted expected return on stocks. In managing a portfolio, market participants compare expected rates of return and select financial assets with the highest expected return. Since stocks and bonds are perfect substitutes in investors' portfolio, the demand for stocks will rise, tending to raise stock prices.
| S |
| D |
| S2 |
| S1 |
6% 6%
| surplus |
| D |
Loanable funds Quantity of bonds
| S |
| D2 |
| D1 |
1333.33 6%
1000.00 8%
Quantity of stocks
Stock prices will rise until the expected return on stocks is again 2 percent higher than the expected return on bonds (4% + 2% = 6%).
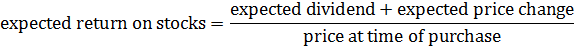
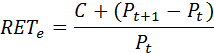
The expected price change by the terms of the example is equal to zero.
Solving for price at the time of purchase:
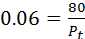 Þ
Þ 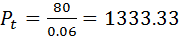
This will occur when the price of share of stock rises to 1333.33 ˆ.
Second, the fall in the interest rate will be expected to raise the demand for goods and services (due to credit expansion) and increase the sales and businesses’ income. With earnings expected to rise, dividends will also be expected to rise. This reinforces the first effect.
For example, if the dividend is expected to rise from 80 to 90 ˆ per share, the price per share increases to 1500 ˆ because 90 ˆ divided by 1500 ˆ is equal to 6 percent (90/1500 = .06 ≈ 6 %).
Again, after stock prices have adjusted to the change in interest rates, the risk-adjusted return on stocks will be equal to the risk-adjusted return on bonds:
6% (return on stocks) – 2% (risk premium) = 4% (return on bonds)
risk-adjusted return on stocks risk-adjusted return on bonds
Date: 2014-12-22; view: 1843
| <== previous page | | | next page ==> |
| THE BENEFITS OF DIVERSIFICATION | | | FORMING OF THE PRICE EXPECTATIONS |