
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
Newton and Leibniz
I. Before you read: Are the names of Newton and Leibniz well known to students of mathematics? What do you know about them?
II. Judging from the title, how will the article be structured? Look through it quickly to see if your prediction was correct.
The essential insight of Newton and Leibniz was to use Cartesian algebra to synthesize the earlier results and to develop algorithms that could be applied uniformly to a wide class of problems. The formative period of Newton's researches was from 1665 to 1670, while Leibniz worked a few years later, in the 1670s. Their contributions differ in origin, development, and influence, and it is necessary to consider each man separately.
The son of an English farmer, Newton became in 1669 the Lucasian professor of mathematics at Cambridge University. Newton's earliest researches in mathematics grew in 1665 from his study of van Schooten's edition of La Géométrie and Wallis' Arithmetica Infinitorum. Using the Cartesian equation of the curve, he reformulated Wallis' results, introducing for this purpose infinite sums in the powers of an unknown x, now known as infinite series. Possibly under the influence of Barrow, he used infinitesimals to establish for various curves the inverse relationship of tangents and areas. The operations of differentiation and integration emerged in his work as analytic processes that could be applied generally to investigate curves.
Unusually sensitive to questions of rigour, Newton at a fairly early stage tried to establish his new method on a sound foundation using ideas from kinematics. A variable was regarded as a “fluent,” a magnitude that flows with time; its derivative or rate of change with respect to time was called a “fluxion,” denoted by the given variable with a dot above it. The basic problem of the calculus was to investigate relations among fluents and their fluxions. Newton finished a treatise on the method of fluxions as early as 1671, although it was not published until 1736. In the 18th century this method became the preferred approach to the calculus among British mathematicians, especially after the appearance in 1742 of Colin Maclaurin's influential Treatise of Fluxions.
Newton first published the calculus in Book I of his great Philosophiae Naturalis Principia Mathematica (1687) (Mathematical Principles of Natural Philosophy). Originating as a treatise on the dynamics of particles, the Principia presented an inertial physics that combined Galileo's mechanics and Kepler's planetary astronomy. It was written in the early 1680s at a time when Newton was reacting against Descartes's science and mathematics. Setting aside the analytic method of fluxions, Newton introduced in 11 introductory lemmas his calculus of first and last ratios, a geometric theory of limits that provided the mathematical basis of his dynamics.
Newton's use of the calculus in the Principia is illustrated by proposition 11 of Book I: If the orbit of a particle moving under a centripetal force is an ellipse with the centre of force at one focus, then the force is inversely proportional to the square of the distance from the centre. Because the planets were known by Kepler's laws to move in ellipses with the Sun at one focus, this result supported his inverse square law of gravitation. To establish the proposition, Newton derived an approximate measure for the force by using small lines defined in terms of the radius (the line from the force centre to the particle) and the tangent to the curve at a point. This result expressed geometrically the proportionality of force to vector acceleration. Using properties of the ellipse known from classical geometry, Newton calculated the limit of this measure and showed that it was equal to a constant times one over the square of the radius.
Newton avoided analytical processes in the Principia by expressing magnitudes and ratios directly in terms of geometric quantities, both finite and infinitesimal. His decision to eschew analysis constituted a striking rejection of the algebraic methods that had been important in his own early researches on the calculus. Although the Principia was of inestimable value for later mechanics, it would be reworked by researchers on the Continent and expressed in the mathematical idiom of the Leibnizian calculus.
Leibniz's interest in mathematics was aroused in 1672 during a visit to Paris, where the Dutch mathematician Christiaan Huygens introduced him to his work on the theory of curves. Under Huygens' tutelage Leibniz immersed himself for the next several years in the study of mathematics. He investigated relationships among the summing and differencing of finite and infinite sequences of numbers. Having read Barrow's geometric lectures, he devised a transformation rule to calculate quadratures, obtaining the famous infinite series for π/4:
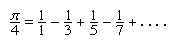
Leibniz was interested in questions of logic and notation, of how to construct a characteristica universalis for rational investigation. After considerable experimentation he arrived by the late 1670s at an algorithm based on the symbols d and ∫. He first published his research on differential calculus in 1684 in an article in the Acta Eruditorum, “A New Method for Maxima and Minima as Well as Tangents, Which Is Impeded Neither by Fractional nor by Irrational Quantities, and a Remarkable Type of Calculus for This”. In this article he introduced the differential dx satisfying the rules d(x + y) = dx + dy and d(xy) = xdy + ydx and illustrated his calculus with a few examples. Two years later he published a second article, “On a Deeply Hidden Geometry,” in which he introduced and explained the symbol ∫ for integration. He stressed the power of his calculus to investigate transcendental curves, the very class of “mechanical” objects Descartes had believed lay beyond the power of analysis, and derived a simple analytical formula for the cycloid.
Leibniz continued to publish results on the new calculus in the Acta Eruditorum and began to explore his ideas in extensive correspondence with other scholars. Within a few years he had attracted a group of researchers to promulgate his methods, including the brothers Johann and Jakob Bernoulli in Basel and the priest Pierre Varignon and Guillaume-François-Antoine de L'Hospital in Paris. In 1700 he convinced Frederick III of Prussia to establish the Brandenburg Society of Sciences (later renamed the Berlin Academy of Sciences), with himself appointed president for life.
Leibniz's vigorous espousal of the new calculus, the didactic spirit of his writings, and his ability to attract a community of researchers contributed to his enormous influence on subsequent mathematics. In contrast, Newton's slowness to publish and his personal reticence resulted in a reduced presence within European mathematics. Although the British school in the 18th century included capable researchers, Abraham De Moivre, James Stirling, Brook Taylor, and Maclaurin among them, they failed to establish a program of research comparable to that established by Leibniz's followers on the Continent. There is a certain tragedy in Newton's isolation and his reluctance to acknowledge the superiority of continental analysis. As the historian Michael Mahoney observed:
Whatever the revolutionary influence of the Principia, mathematics would have looked much the same if Newton had never existed. In that endeavour he belonged to a community, and he was far from indispensable to it.
III. Analyse the cases of the use of Participles in the passage and translate the sentences containing them.
IV. How will you translate the Syntactical Constructions in the sentences:
1. In 1700 he convinced Frederick III of Prussia to establish the Brandenburg Society of Sciences (later renamed the Berlin Academy of Sciences), with himself appointed president for life.
2. Because the planets were known by Kepler's laws to move in ellipses with the Sun at one focus, this result supported his inverse square law of gravitation.
V. How do you understand the sentence:
Newton calculated the limit of this measure and showed that it was equal to a constant times one over the square of the radius.
VI. Some words are difficult to translate. Look up the meanings of the italicized words in the dictionary and translate the sentences:
1. The essential insight of Newton and Leibniz was to use Cartesian algebra…
2. Their contributions differ in origin, development, and influence, and it is necessary to consider each man separately.
3. Newton at a fairly early stage tried to establish his new method on a sound foundation…
4…it would be reworked by researchers on the Continent and expressed in the mathematical idiom of the Leibnizian calculus.
VII. The word "establish" is used repeatedly in the passage. Do you think it is used in the same meaning? Compare the contexts and present the translation of the sentences:
1.…he used infinitesimals to establish for various curves the inverse relationship of tangents and areas.
2.…Newton at a fairly early stage tried to establish his new method on a sound foundation using ideas from kinematics
3. To establish the proposition, Newton derived an approximate measure for the force by using small lines defined in terms of the radius (the line from the force centre to the particle) and the tangent to the curve at a point.
4. In 1700 he convinced Frederick III of Prussia to establish the Brandenburg Society of Sciences (later renamed the Berlin Academy of Sciences)…
5.…they failed to establish a program of research comparable to that established by Leibniz's followers on the Continent.
VIII. Work in pairs: Student A reads the part about Newton, Student B about Leibniz. Pick out the information about Newton's and Leibniz's contributions to mathematics and get ready to present it to your partner.
IX. Can you prove that Newton's and Leibniz's contributions differ in origin, development, and influence?
What are the reasons for that?
X. Do you agree with the estimate given to Newton in the concluding lines of the article?
…mathematics would have looked much the same if Newton had never existed.
XI. Fill in the missing forms:
| Noun | Verb | Adjective | Adverb |
| differentiation | |||
| reformulate | |||
| proposition | |||
| analytic | |||
| mathematics | |||
| investigate | |||
| approximately | |||
| experimentation | |||
| revolutionary |
XII. Match the words with their antonyms:
finite unknown
appearance incapable
inestimable approximate
synthetic infinite
capable disappearance
exact estimable
known analytic
XIII. Suggest the Russian counterparts for the following words and expressions:
to develop algorithms, an infinite sum, an infinite series, under the influence of, the inverse relationship, an infinitesimal, to establish relationship, operations of differentiation and integration, to establish a new method, a variable, a rate of change, with respect to, an inertial physics, calculus of first and last ratios, the theory of limits, provided the basis of, to move under a centripetal force, to be inversely proportional to, an inverse square law , an approximate measure, in terms of, geometric quantities, the theory of curves, finite and infinite sequences of numbers, to arrive at an algorithm, differential calculus, to satisfy a rule, transcendental curves, to derive a formula, to contribute to, in contrast, to result in, comparable to, to acknowledge the superiority.
Date: 2015-01-29; view: 1512
| <== previous page | | | next page ==> |
| Institutional background | | | The 18th century |