
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
The fundamental law of mechanics
Second law. This law is basic of classical mechanics. The acceleration of a body is directly proportional to the vector sum of forces acting upon it and is inversely proportional to the mass of the body. The direction of acceleration coinsides with direction of the force sum.
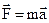 (4.16)
(4.16)
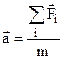
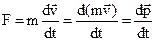 (4.17)
(4.17)
The derivative of impulse with respect to time equals to the vector sum of forces, acted upon the body. If the forces 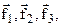
 , whose sum total is
, whose sum total is
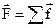 (4.18)
(4.18)
act on a body, the acceleration acquired by the bodies equals to the quotient by dividing the resultant force by the

Figure 4.4
The law of inertia follows directly from the fundamental law. If there are no forces acting on the body, the acceleration is equal to zero and the motion of the body is rectilinear and uniform.
First law. Everybody retains its state of rest or straight line uniform motion unless its compelled to change that state by the external forces. Possibility of body to be in a state of rest or of uniform rectilinear (straight line) motion is called inertia and so 1st Newton’s Law is the law of inertia.
In applying Newton’s fundamental law to a particular body, we focus our attention on this body and consider the forces acting on it. It should not be forgotten, however, that force is a measure of the interaction between bodies and that one-sided interaction does not exist. If one body acts on another, the latter also acts on the former. The measurement of force is equivalent to the measurement of interaction. Thus, the very method of measuring force assumes that the force of one body acting on another and the force exerted by the latter on the former are equivalent in magnitude. Since we are usually interested in one particular body, we focus our attention on the force acting on it; the other force is called the force of counteraction of the force of reaction. The forces of action and reaction are equal in magnitude but are oppositely directed. This proposition has become known as Newton’s third law of motion.
Third law of motion: “To every action there is always opposed an equal reaction: or, the mutual actions of two bodies upon each other are always equal, and directed to contrary parts”
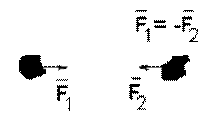
Figure 4.5
The forces with which two bodies act upon each other are equal in magnitude and opposite in direction.
 (4.19)
(4.19)
Newton’s laws are correct only if:
1) a = 0. The coordinate system does not accelerate. This coordinate system are called inertial.(frame of reference).
2) Body dimension are much more than atomic ones.
3) The body velocity is much less than the velocity of light v << c .
The system for which Newton’s laws are valid must, without fail, satisfy the following conditions: a body on which no forces are acting must move rectilinearly and uniformly or must be at rest. Such a system is called an inertial system.
The principle of the relativity of motion: an infinite number of inertial system exist and, in such systems, the law of inertia and the 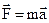 law are satisfied. In this respect, none of these systems has any special advantage over the other systems. All inertial systems are equally suitable for the description of physical phenomena.
law are satisfied. In this respect, none of these systems has any special advantage over the other systems. All inertial systems are equally suitable for the description of physical phenomena.
Date: 2015-01-12; view: 1289
| <== previous page | | | next page ==> |
| Gravitational force | | | Motion in circle is accelerated motion |