Module 2. Analitic geometry. Introduction to Calculus

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 1

1. Calculate the limits: а) ; b) ;

c) ; d) .

2. Calculate the limits: а) ; b) . .

3 Investigate the function for continuity: .

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 2

1. Calculate the limits: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity.

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 3

1Calculate the limits: а) ; b) ;

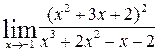c) ; d) .

4. Calculate the limits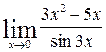: а) ; b) . .

5. Investigate the function for continuity: .

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 4

1. Calculate the limits: а) ; b) ;

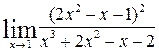c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: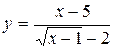

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 5

1.

Calculate the limits: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: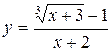

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 6

1.. Calculate the limits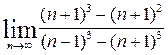: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: .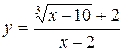

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 7

1.. Calculate the limits: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: .

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 8

Calculate the limits: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: .

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 9

1Calculate the limits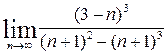: а) ; b) ;

c) ; d) .

4. Calculate the limits: а) ; b) .

5. Investigate the function for continuity: .

Module 2. Analitic geometry. Introduction to Calculus

Individual Home Task

Variant 10

1. Calculate the limits: а) ; b) ;

c) ; d) .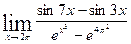

5. Calculate the limits: а) ; b) .

6. Investigate the function for continuity: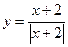

Module 2. Analitic geometry. Introduction to Calculus

Date: 2015-12-24; view: 1060