
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
Relation and set functions.
The relation from the set A in the set B 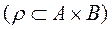 is called the function from A into B, if
is called the function from A into B, if
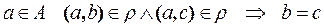 .
.
The fact that f function from the set A into the set B is registered as f: 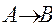 or
or 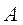

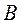 .
.
Instead of the record 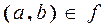 we use
we use  . Such property of the relation is called as uniqueness or functionality.
. Such property of the relation is called as uniqueness or functionality.
If b=f(a) then a is called the argument, and b the function value.
Let
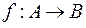 , then
, then 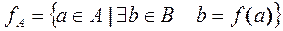 is called the function of the definition range.
is called the function of the definition range.
Let
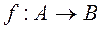 , then
, then 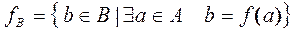 is called the area of function values.
is called the area of function values.
If 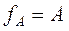 , that function is called the total, and if
, that function is called the total, and if 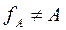 the partial.
the partial.
The narrowing of the set function 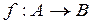 into the set
into the set  is called the function narrowing,
is called the function narrowing, 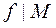 ,it is defined as follows:
,it is defined as follows:
 .
.
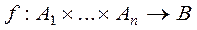 is called the function of n arguments or n local function.
is called the function of n arguments or n local function.
Properties of function: Let 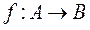 . Then function
. Then function 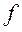 is called the injective, if
is called the injective, if
 , the subjective, if
, the subjective, if
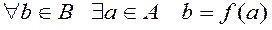 , objective, if it injective and subjective
, objective, if it injective and subjective
Properties of the relations
Let  , i.e.
, i.e. 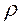 the binary relation on the set A
the binary relation on the set A
1) 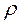 reflexive relation, if
reflexive relation, if 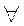

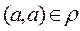
It is read as: for any element from the set À the steam (à, à) belongs to the relation 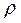 that means that any element from the set À is in the relation with itself.
that means that any element from the set À is in the relation with itself.
For example, we will consider the relation r={(a,b)}½a is a group mate of b,
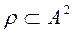 , A great number of students of technical school, then condition
, A great number of students of technical school, then condition 

 means that any student of technical school is a groupmate of himself that obviously isn't correct, it means that the relation
means that any student of technical school is a groupmate of himself that obviously isn't correct, it means that the relation 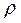 isn't reflexive.
isn't reflexive.
Let's consider the relation 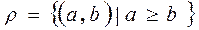 ,
,  , A set of all real numbers, then condition
, A set of all real numbers, then condition 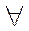
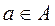
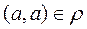 means that any real number is more or equal to itself
means that any real number is more or equal to itself 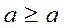 , it is obviously correct, it means that the relation
, it is obviously correct, it means that the relation 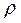 is reflexive.
is reflexive.
2) 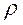 the symmetric relation, if
the symmetric relation, if 

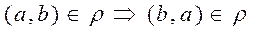 .
.
It is read as follows: for any elements from the set À, if the pair  belongs to the relation
belongs to the relation 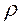 and the pair
and the pair 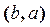 belongs to the relation
belongs to the relation  , that means that if an element a is in relation with b, then element b is in relation with a.
, that means that if an element a is in relation with b, then element b is in relation with a.
Let's consider the relation r={(a,b)}½a is a groupmate of b, 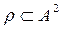 , A great number of students of technical school, then condition
, A great number of students of technical school, then condition 
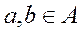
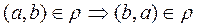 means that if the student of technical school a is the groupmate of the student b, the student b studies in one group with a, it is obviously correct, the relation
means that if the student of technical school a is the groupmate of the student b, the student b studies in one group with a, it is obviously correct, the relation 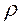 is symmetric.
is symmetric.
Let's consider the relation
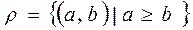 ,
, 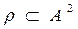 , Aset of all real numbers, then condition
, Aset of all real numbers, then condition 

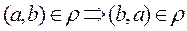 means that if the condition
means that if the condition  is implemented, the condition
is implemented, the condition 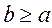 is implemented too, that isn't correct, the relation
is implemented too, that isn't correct, the relation 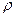 isn't the symmetric.
isn't the symmetric.
3)  antisymmetric relation, if
antisymmetric relation, if 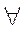
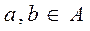
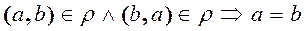 .
.
It is read as follows: for any elements from the set A, if the pair  belongs to the relation
belongs to the relation 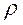 and the pair
and the pair  belongs to the relation
belongs to the relation  , then
, then  , that means that the relation
, that means that the relation 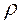 can't contain the pair
can't contain the pair  at the same time with the pair
at the same time with the pair 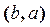 , if the element a is distinct from an element b.
, if the element a is distinct from an element b.
Let's consider the relation  ,
, 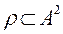 , A set of all real numbers, then condition
, A set of all real numbers, then condition 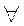
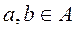
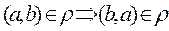 means that if the conditions
means that if the conditions 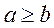 and
and 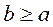 are implemented, the condition
are implemented, the condition 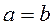 is implemented too, it means that the relation
is implemented too, it means that the relation  is antisymmetric.
is antisymmetric.
Let's consider the relation  ,
, 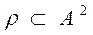 , A set of all real numbers, then the condition
, A set of all real numbers, then the condition 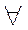

 means that if conditions
means that if conditions  and
and 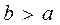 are implemented, the condition
are implemented, the condition 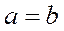 is implemented too, that isn't correct, it means that the relation
is implemented too, that isn't correct, it means that the relation 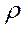 isn't antisymmetric.
isn't antisymmetric.
4) 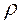 transitive relation, if
transitive relation, if
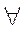
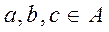
 . It is read as follows: for any elements from the set A, if the pair
. It is read as follows: for any elements from the set A, if the pair 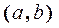 belongs to the relation
belongs to the relation 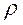 , the pair
, the pair 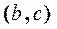 belongs to the relation
belongs to the relation 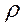 and the pair
and the pair 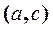 belongs to the relation
belongs to the relation 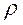 , it means that if the element a is in the relation with b and the element b is in relation with c, then the element a is in relation with c.
, it means that if the element a is in the relation with b and the element b is in relation with c, then the element a is in relation with c.
Let's consider the relation r={(a,b)}½a is a groupmate of b,  , A great number of students of technical school, then condition
, A great number of students of technical school, then condition 

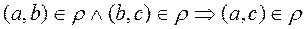 means that if the student of technical school a studies in one group with b and student b studies in one group with ñ, then student à studies in one group with ñ, that is obviously correct, means, the relation
means that if the student of technical school a studies in one group with b and student b studies in one group with ñ, then student à studies in one group with ñ, that is obviously correct, means, the relation 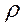 is transitive.
is transitive.
5) 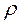 complete or linear relation, if
complete or linear relation, if

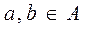
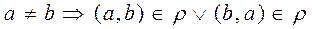 .
.
It is read as follows: for any element from the set À, if 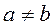 , then the pair
, then the pair 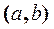 belongs to the relation
belongs to the relation 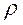 , that means that for any two different elements a is in the relation with b or the element b is in relation with a .
, that means that for any two different elements a is in the relation with b or the element b is in relation with a .
Let's consider the relation
r={(a,b)}½a is a groupmate of b,  ,
,
A great number of students of technical school, then condition
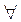

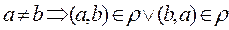 means that for any two students of technical school a and b, or student a is the groupmate of the student b or student b is the groupmate of the student a, that obviously it is not true, it means that the relation
means that for any two students of technical school a and b, or student a is the groupmate of the student b or student b is the groupmate of the student a, that obviously it is not true, it means that the relation 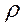 isn't full.
isn't full.
Let's consider the relation 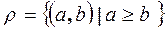 ,
, 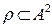 ,
,
A set of all real numbers, then condition
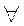
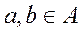
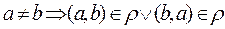 means that for any two various numbers a and b or the condition
means that for any two various numbers a and b or the condition 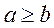 is implemented or the condition
is implemented or the condition 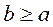 is implemented, that, truly, means, the relation
is implemented, that, truly, means, the relation 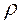 is full.
is full.
Functions
The relation from the set A into the set B 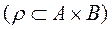 is called the function from À into Â, if
is called the function from À into Â, if
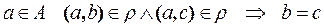 .
.
The fact that f function from the set A into the set B is registered as f: 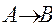 or
or 

 .
.
Instead of record 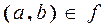 let's use
let's use  . Such property of the relation is called unambiguity or functionality.
. Such property of the relation is called unambiguity or functionality.
If  then
then 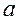 is an argument and
is an argument and  is a value of function.
is a value of function.
Let 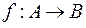 , then
, then 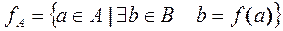 is called the function range of definition.
is called the function range of definition.
Let 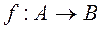 , then
, then 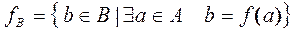 is called the area of values of function.
is called the area of values of function.
If 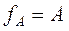 , then the function is called as total and if
, then the function is called as total and if 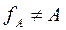 the function is partial.
the function is partial.
Function narrowing 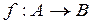 on the set
on the set  is called
is called 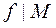 ,it is defined as follows:
,it is defined as follows:  .
. 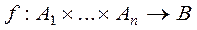 is called the function of n arguments or n local function.
is called the function of n arguments or n local function.
Properties of the function
Let 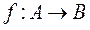 . Then function f is called injective, if
. Then function f is called injective, if  , then the function F is subjective, if
, then the function F is subjective, if
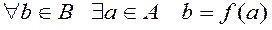 , if it is injective and subjective.
, if it is injective and subjective.
Concept of the set
The concept of the set is one of the main concepts of mathematics. It has no exact definition and has, as a rule, it is explained with the help of examples.
Let's make the following intuitive definition of concept of the set:
Set a certain set of objects. Objects of the set are called the set elements.
For example. The set of houses in one street, a set of natural numbers, a great number of students of group etc.
Sets are usually designated by capital Latin letters À, Â, Ñ, D, X, Y , set elements are determined by small Latin letters a, b, c, d, x, y
To designate the fact that the object x is a set element of A, we use the following symbols: x 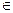 À (it is read as: x belongs to À), x
À (it is read as: x belongs to À), x 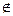 À designates that the object x isn't a set element of A (it is read as: x doesn't belong to À).
À designates that the object x isn't a set element of A (it is read as: x doesn't belong to À).
The set not containing any element is called an empty set (it is designated as Ø).
The sets with elements of which we make a concrete set is called the universal set (it is designated as U).
For example. U great number of people on the earth, À students of group E-102.
Sets can be represented by means of circles which are called Euler's circles, the universal set can be designated a rectangle.
Ways of presentation of sets
To establish the set, it is necessary to specify, what elements belong to it. It can be done in the various ways: 1) Enumeration of all elements of the set in braces.
Example: A= {Astana, Atbasar, Karaganda}
2) A characteristic predicate which describes the property of all elements entering into the set. The characteristic predicate is registered after the colon or the symbol « |».
For example. Ð(x) = x 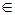 N
N  x < 8 characteristic predicate.
x < 8 characteristic predicate.
M = {x: Ð(x)} or M = {x: x 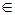 N
N  x < 8}. The set M can be represented the enumeration of its elements: M = {1, 2, 3, 4, 5, 6, 7}
x < 8}. The set M can be represented the enumeration of its elements: M = {1, 2, 3, 4, 5, 6, 7}
For example. Â = {x | x natural number} = {2, 4, 6, 8, }
If the set consists of a small amount of elements, it is convenient to represent it by enumeration of all elements, if there are a lot of elements or the set has infinite number of elements, it is established by means of the characteristic predicate.
The following numerical sets are known from the school course: N set of natural numbers, N = {1, 2, 3, 4, }; Z set of integers, Z = { , 3, 2, 1, 0, 1, 2, 3, 4, }; Q set of rational numbers,
Date: 2015-12-24; view: 3463
| <== previous page | | | next page ==> |
| Information representation options in the Computer | | | Bases of logic, logician of statements, logic sheaves, validity tables. |