
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
Controlling for the market
However, one may suggest that a high or low return can arise if generally investors are buying or selling stocks on the day. The reason for that might be an economy-wide news piece. Therefore, to identify the abnormal company-related returns we also subtract S&P 500 index return from the stock return. Then we shall have a return of the company  less the overall investors’ buying attitude
less the overall investors’ buying attitude  – the adjusted company return
– the adjusted company return  .
.
This would make more sense as it removes market-wide news. Moreover, most of the sampled stocks exhibit appreciation during the sample period, which means that a negative return is less likely to happen than positive (or that the positive returns’ scale is larger), therefore even if a piece of news led to significant positive return for company A (say, 7%) it may only make its competitor to grow slower than usual (say, 0.2% instead of 1.2%). S&P 500 index itself grew by 44% during the sample period and if we subtract it from the company returns we may identify the “losers” and “winners” as well as “better than index”, or positive returns and “worse than index”, or negative returns. In the previous case, say the S&P rose by 2.5%, then company A performed (7-2.5)=4.5% better than the index, while its competitor performed (0.2-2.5)=-2.2% worse than the index. After subtraction it is easier to see whether a news article affected both the company A and its competitor.
| Date | INITIAL RETURNS | ADJUSTED | |||||
| AGILENT TECHS. | TERADYNE | THERMO FISHER SCIENTIFIC | S&P 500 | AGILENT TECHS. | TERADYNE | THERMO FISHER SCIENTIFIC | |
| 28/11/2011 | 0.039 | 0.036 | 0.026 | 2.88% | 0.97% | 0.73% | -0.26% |
| 29/11/2011 | -0.004 | -0.002 | 0.011 | 0.22% | -0.62% | -0.47% | 0.85% |
| 30/11/2011 | 0.068 | 0.108 | 0.025 | 4.24% | 2.60% | 6.58% | -1.75% |
| 01/12/2011 | 0.008 | -0.007 | -0.004 | -0.19% | 1.04% | -0.48% | -0.25% |
| 02/12/2011 | -0.028 | -0.016 | -0.011 | -0.02% | -2.82% | -1.56% | -1.04% |
| 05/12/2011 | 0.008 | 0.025 | 0.005 | 1.02% | -0.21% | 1.45% | -0.51% |
| 06/12/2011 | -0.002 | 0.009 | 0.009 | 0.11% | -0.33% | 0.78% | 0.74% |
| 07/12/2011 | -0.009 | 0.001 | -0.004 | 0.20% | -1.07% | -0.05% | -0.63% |
| 08/12/2011 | -0.063 | -0.019 | -0.037 | -2.14% | -4.17% | 0.29% | -1.53% |
| 09/12/2011 | 0.026 | 0.054 | 0.008 | 1.67% | 0.94% | 3.71% | -0.90% |
| 12/12/2011 | -0.046 | -0.029 | -0.011 | -1.50% | -3.13% | -1.37% | 0.38% |
| 13/12/2011 | -0.017 | -0.034 | -0.01 | -0.87% | -0.86% | -2.54% | -0.11% |
| 14/12/2011 | -0.007 | -0.029 | -0.021 | -1.14% | 0.48% | -1.77% | -0.98% |
| 15/12/2011 | 0.016 | -0.001 | 0.009 | 0.32% | 1.24% | -0.40% | 0.59% |
| Table 2 – Adjusting data for market returns |
The “Adjusted” part of the table (table 2) represents companies’ daily returns less S&P return. On 28th of November, 2011 all three companies showed to have exceptional returns. However, the S&P index itself showed a return of 2.88% on that day as well and therefore we may not conclude that all three stocks had positive news on that day. After the adjustments the companies show relative returns ranging from -0.26% to 0.97%, which are not that large. However, not all events are hidden with the adjustments. On 8th of December, 2011 (table 4) we see that even after the subtraction of S&P’s return Agilent Technologies stock has a significant negative return of -4.7% and other outliers still remain.
However, comparing the adjusted returns themselves is not comfortable. Some returns are negative and some are positive. So I first take the square of the returns, and then find the outliers. Squaring the returns will magnify the heteroscedastic properties of the returns as well as make it easier to compare negative and positive returns.
| Graph 3 – Agilent Technologies squared returns |

Ratio Analysis
We find outliers in the returns by using the formula 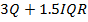 . The 3rd quartile represents an observation which is larger than three quarters of all of the observations. The IQR (Inter-Quartile range) is the difference between the third quartile and the first quartile, where the 1st quartile represents an observation which is larger than one quarter of all of the observations. If the adjusted company A’s return satisfies the following inequality, it is considered an outlier:
. The 3rd quartile represents an observation which is larger than three quarters of all of the observations. The IQR (Inter-Quartile range) is the difference between the third quartile and the first quartile, where the 1st quartile represents an observation which is larger than one quarter of all of the observations. If the adjusted company A’s return satisfies the following inequality, it is considered an outlier:

We only look for outliers on the upper side (not 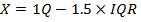 ), because all of our returns are squared and therefore nonnegative, while the lower critical value of X is below 0. For all of the stocks the number of days is 503, representing two trading years. Out of these days, there are approximately 50±5 outliers for different companies. These outliers represent a significant difference in the returns between the stock and the S&P 500 index.
), because all of our returns are squared and therefore nonnegative, while the lower critical value of X is below 0. For all of the stocks the number of days is 503, representing two trading years. Out of these days, there are approximately 50±5 outliers for different companies. These outliers represent a significant difference in the returns between the stock and the S&P 500 index.
To see, whether the competitors’ stock prices are affected I am going to do the following tests:
First is to see, whether a significant return in company A’s stock causes a significant opposite return in the competitors’ stock prices. To do so, I first identify the outliers for company A and its competitors. Then, I check, if outliers of both companies coincide, which we call co-reactions. Finally, I check whether the outliers have opposite signs. The number of these events is calculated and divided by the number of outliers in the returns of company A, which provides us with the ratio RN (standing for co-Reactions Negative). The proportion is tested against the null hypothesis of being less or equal to the Opposite Ratio (OR). The opposite ratio is the number of times the company A had opposite returns with its competitors over the total number of observations. If the RN ratio is higher than the OR ratio we can say that significant news on average has a significant opposite effect on the competitors’ share prices.
Secondly; I am going to check if a significant return for company A causes a general opposite return in the competitors’ stock (not necessarily significant). This means checking if an outlier in the company A’s returns coincides with an opposite return in the competitors’ stocks on the same day. The number of such events is calculated, divided by the total number of outliers in company A’s return and named Neg (Negative ratio). The Neg ratio is then tested against the null hypothesis of being less or equal to the Opposite Ratio. If the null hypothesis is rejected we can conclude that significant news for company A has some opposite effect on the competitors’ share prices.
This test is run because the first test did not show significant opposite effect on competitors. So the goal is to check whether there is any negative effect at all.
The table below shows the how adjusted returns are calculated. The control returns are subtracted from the Initial returns, which gives the adjusted returns. For Agilent Technologies (US:A), the adjuster returns are:  . The same process is done for Teradyne (US:TER) and Thermo Fisher Scientific (TMO). Then the adjusted returns are squared and outliers are located. The “Outlier Test” part of the table checks if the return at time t fits the outlier inequality. If that is the case “TRUE” value is displayed.
. The same process is done for Teradyne (US:TER) and Thermo Fisher Scientific (TMO). Then the adjusted returns are squared and outliers are located. The “Outlier Test” part of the table checks if the return at time t fits the outlier inequality. If that is the case “TRUE” value is displayed.
| Date | Initial Returns | Control | Adjusted Returns | Outlier Test | ||||||
| A | TER | TMO | SP500 | A | TER | TMO | A | TER | TMO | |
| OUTLIER? | OUTLIER? | OUTLIER? | ||||||||
| 28/11/2011 | 3.90% | 3.60% | 2.60% | 2.88% | 0.97% | 0.73% | -0.26% | |||
| 29/11/2011 | -0.40% | -0.20% | 1.10% | 0.22% | -0.62% | -0.47% | 0.85% | |||
| 30/11/2011 | 6.80% | 10.80% | 2.50% | 4.24% | 2.60% | 6.58% | -1.75% | TRUE | TRUE | TRUE |
| 01/12/2011 | 0.80% | -0.70% | -0.40% | -0.19% | 1.04% | -0.48% | -0.25% | |||
| 02/12/2011 | -2.80% | -1.60% | -1.10% | -0.02% | -2.82% | -1.56% | -1.04% | TRUE | ||
| 05/12/2011 | 0.80% | 2.50% | 0.50% | 1.02% | -0.21% | 1.45% | -0.51% | |||
| 06/12/2011 | -0.20% | 0.90% | 0.90% | 0.11% | -0.33% | 0.78% | 0.74% | |||
| 07/12/2011 | -0.90% | 0.10% | -0.40% | 0.20% | -1.07% | -0.05% | -0.63% | |||
| 08/12/2011 | -6.30% | -1.90% | -3.70% | -2.14% | -4.17% | 0.29% | -1.53% | TRUE | TRUE | |
| 09/12/2011 | 2.60% | 5.40% | 0.80% | 1.67% | 0.94% | 3.71% | -0.90% | TRUE | ||
| 12/12/2011 | -4.60% | -2.90% | -1.10% | -1.50% | -3.13% | -1.37% | 0.38% | TRUE | ||
| 13/12/2011 | -1.70% | -3.40% | -1.00% | -0.87% | -0.86% | -2.54% | -0.11% | TRUE | ||
| 14/12/2011 | -0.70% | -2.90% | -2.10% | -1.14% | 0.48% | -1.77% | -0.98% | |||
| 15/12/2011 | 1.60% | -0.10% | 0.90% | 0.32% | 1.24% | -0.40% | 0.59% | |||
| 16/12/2011 | 0.00% | 2.50% | -0.70% | 0.32% | -0.29% | 2.21% | -1.03% | |||
| 19/12/2011 | -0.70% | -3.00% | -0.70% | -1.18% | 0.49% | -1.82% | 0.49% | |||
| 20/12/2011 | 4.00% | 5.70% | 3.10% | 2.94% | 1.04% | 2.75% | 0.20% | TRUE | ||
| 21/12/2011 | -0.60% | -1.00% | -0.60% | 0.19% | -0.83% | -1.23% | -0.80% |
| Table 3 – Testing returns for abnormal volatility, denoted as “outliers” A – Agilent Technologies, TER – Teradyne, TMO – Thermo Fisher Scientific SP500 – Standard & Poor 500 |
For the first test we only look for cases, when company A’s outliers coincide with its competitors’ outliers. Therefore we filter and leave only “true” values for the company (Agilent Technologies, A). However, a case when there are two outliers does not mean that a significant return of Agilent Technologies led to a significant opposite return for its competitors. As we can see, on the 8th of December Agilent Technologies and Thermo Fisher Scientific both had significant negative returns and therefore we cannot use that day for our first test. To satisfy the first test we need two things to be true: there are significant returns for 2 (or three) companies AND the returns are opposite for the company A and its competitors.
The “Reaction?” table checks whether the values of “TRUE” coincide for A-TER (Agilent Technologies and Teradyne) and for A-TMO (Agilent Technologies and Thermo Fisher Scientific). In other words it checks if outliers happen on the same day for two companies. The “Opposite?” table checks whether the returns for the pair of stock are of opposite sign. Finally the “R and N” table checks if both the “Reaction?” and “Opposite?” tables say “Yes”, that is if a significant return for Agilent Technologies coincides with a significant opposite return of its competitor.
| Date | Opposite test | Outlier test | co-Reaction test | RN test | |||||
| A-TER | A-TMO | A | TER | TMO | A-TER | A-TMO | A-TER | A-TMO | |
| Opposite? | Opposite? | Outlier? | Outlier? | Outlier? | Reaction? | Reaction? | R and N | R and N | |
| 30/11/2011 | TRUE | TRUE | TRUE | ||||||
| 02/12/2011 | TRUE | ||||||||
| 08/12/2011 | TRUE | TRUE | |||||||
| 12/12/2011 | TRUE | ||||||||
| 28/12/2011 | TRUE | TRUE | |||||||
| 03/01/2012 | TRUE | TRUE | |||||||
| 09/01/2012 | TRUE | ||||||||
| 17/01/2012 | TRUE | ||||||||
| 18/01/2012 | TRUE | TRUE | TRUE | ||||||
| 20/01/2012 | TRUE | ||||||||
| 24/01/2012 | TRUE | ||||||||
| 25/01/2012 | TRUE | ||||||||
| 26/01/2012 | TRUE | TRUE | TRUE | ||||||
| 01/02/2012 | TRUE | TRUE | |||||||
| 03/02/2012 | TRUE | ||||||||
| 07/03/2012 | TRUE | TRUE | |||||||
| 12/03/2012 | TRUE | TRUE | |||||||
| 05/04/2012 | TRUE |
| Table 4 – Testing for opposite co-Reactions A – Agilent Technologies, TER – Teradyne, TMO – Thermo Fisher Scientific SP500 – Standard & Poor 500 |
Then I do the second test, which checks if a significant return in the stock A happens with a general opposite (not necessarily significant) return in the competitors’ stock. Table “Neg” checks if an outlier in Agilent Technologies’ adjusted returns coincides with an opposite return in competitor’s stock.
| Date | Opposite test | Outlier test | Negative test | ||||
| A-TER | A-TMO | A | TER | TMO | A-TER | A-TMO | |
| Opposite? | Opposite? | Outlier? | Outlier? | Outlier? | NEG | NEG | |
| 30/11/2011 | TRUE | TRUE | TRUE | ||||
| 02/12/2011 | TRUE | ||||||
| 08/12/2011 | TRUE | TRUE | |||||
| 12/12/2011 | TRUE | ||||||
| 28/12/2011 | TRUE | TRUE | |||||
| 03/01/2012 | TRUE | TRUE | |||||
| 09/01/2012 | TRUE | ||||||
| 17/01/2012 | TRUE | ||||||
| 18/01/2012 | TRUE | TRUE | TRUE | ||||
| 20/01/2012 | TRUE | ||||||
| 24/01/2012 | TRUE | ||||||
| 25/01/2012 | TRUE | ||||||
| 26/01/2012 | TRUE | TRUE | TRUE | ||||
| 01/02/2012 | TRUE | TRUE | |||||
| 03/02/2012 | TRUE | ||||||
| 07/03/2012 | TRUE | TRUE | |||||
| 12/03/2012 | TRUE | TRUE | |||||
| 05/04/2012 | TRUE |
| Table 5 – Testing for opposite returns given significant returns for Agilent Technologies A – Agilent Technologies, TER – Teradyne, TMO – Thermo Fisher Scientific SP500 – Standard & Poor 500 |
The process is continued with all other 28 companies. Then the following ratios are calculated: 1) {the number of times a significant return for company A caused a significant opposite return to its competitors} over {the total number of significant returns for company A} = (Ratio RN) and 2) {the number of times a significant return for company A caused an opposite (not necessarily significant) return to its competitors} over {the total number of significant returns for company A} = (Ratio Neg) 3) {the number of times the company A had opposite returns with its competitors} over {the total number of returns} (Opposite Ratio, OR).
To see if news affect both company A and its competitor we have to compare the ratios RN and Neg with overall “opposite ratio”. If the news do affect both company A and its competitors, then the ratio RN and/or ratio Neg have to be significantly larger than the opposite ratio.
Significant opposite reaction test
H0: news that significantly affects company A does not have a significant opposite effect on its competitors. RN < OR
Ha: news that significantly affects company A has a significant opposite effect on its competitors. RN > OR
Opposite reaction test
H0: news that significantly affects company A does not have an opposite effect on its competitors
Ha: news that significantly affects company A has an opposite effect on its competitors
| Competitor 1 | Competitor 2 | |||||
| OR | RN | Neg | OR | RN | Neg | |
| A | 38.57% | 4.08% | 28.57% | 39.76% | 6.12% | 26.53% |
| AAPL | 45.33% | 5.26% | 54.39%* | 50.70% | 1.75% | 29.82% |
| ADI | 32.60% | 0.00% | 3.70% | |||
| ALTR | 27.83% | 1.82% | 14.55% | |||
| AMAT | 31.81% | 0.00% | 10.71% | |||
| AMD | 41.35% | 10.00% | 30.00% | 53.48% | 8.00% | 52.00% |
| BRCM | 40.76% | 3.28% | 26.23% | 35.98% | 3.28% | 27.87% |
| CIEN | 41.95% | 0.00% | 25.49% | |||
| CSCO | 41.95% | 0.00% | 33.33% | 48.71% | 1.85% | 38.89% |
| EMC | 49.30% | 5.56% | 55.56% | 43.94% | 1.85% | 20.37% |
| GOOG | 42.54% | 6.82% | 36.36% | 45.33% | 2.27% | 40.91% |
| HPQ | 45.13% | 1.85% | 38.89% | |||
| IBM | 45.13% | 1.96% | 41.18% | 48.71% | 5.88% | 43.14% |
| INTC | 41.35% | 9.80% | 41.18% | 43.94% | 0.00% | 43.14% |
| KLAC | 31.81% | 0.00% | 20.83% | |||
| LLTC | 29.03% | 0.00% | 5.36% | 30.22% | 0.00% | 10.71% |
| LXK | 45.33% | 3.08% | 55.38%* | |||
| MSFT | 46.72% | 5.77% | 59.62%** | 48.71% | 5.77% | 51.92% |
| MXIM | 36.78% | 2.44% | 12.20% | 35.19% | 2.44% | 14.63% |
| NCR | 50.10% | 8.70% | 47.83% | 49.50% | 6.52% | 47.83% |
| NTAP | 43.54% | 5.00% | 30.00% | 37.57% | 1.67% | 30.00% |
| QCOM | 43.94% | 0.00% | 33.93% | |||
| QLGC | 43.74% | 3.85% | 36.54% | 45.13% | 1.92% | 48.08% |
| SATM | 34.59% | 5.26% | 29.82% | |||
| TMO | 39.76% | 5.56% | 20.37% | |||
| TXN | 38.97% | 0.00% | 25.93% | |||
| XLNX | 27.83% | 1.64% | 9.84% | |||
| XRX | 44.73% | 7.02% | 28.07% | |||
| YHOO | 46.52% | 0.00% | 34.00% | 45.33% | 2.00% | 40.00% |
| Table 6 – Results || Comparing RN and Neg Ratios to the Opposite Ratio * - significantly larger at 90% confidence level ** - significantly larger at 95% confidence level |
Date: 2015-12-17; view: 865
| <== previous page | | | next page ==> |
| Ratios of response to significant news analysis | | | Competitors’ Influence - Regression |