The Determination of the Interest Rate I

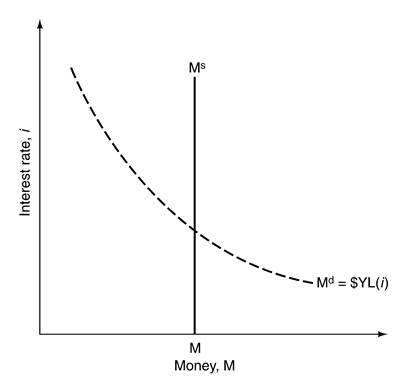
Assume all money is currency, so there are no checking accounts or banks. Consider the supply of money to be fully in the control of the central bank and take nominal income as given. Then, equilibrium in the money market occurs when the supply of money (M) equals the demand for money (M^d) given in equation (4.1). Figure 4.1 illustrates the solution.

An increase in the money supply shifts the vertical line to the right, resulting in a new equilibrium with a lower interest rate. In order to induce the private sector to hold more money, bonds must become less attractive (the interest rate must fall). An increase in nominal income (for a given money supply) shifts the money demand curve to the right and generates a new equilibrium with a higher interest rate. The increase in nominal income increases the demand for money at the original interest rate. Since the supply of money has not changed, something must occur to offset the increase in demand. The increase in the interest rate makes bonds more attractive, thereby reducing the demand for money and offsetting the increase in money demand from the increase in income.

Figure 4.1: Equilibrium in the Money Market

How does the central bank control the money supply? Consider the central bank’s balance sheet. Currency held by the public constitutes the central bank’s liabilities. The central bank’s assets are any bonds that it owns. To increase the money supply, the central bank creates currency to purchase bonds, thus increasing assets (through the additional bonds) and liabilities (through the new currency created and exchanged for bonds). To reduce the money supply, the central bank sells bonds for existing currency, thus reducing assets (through the sale of bonds) and liabilities (through the reduction of currency held by the general public). Purchases and sales of bonds by the central bank are called open market operations.

The text also considers open market operations in terms of their effect on bond prices. Suppose a bond promises a payment of $F in one year. Call the current price of the bond $P_B. Then, the interest rate (or rate of return) on this bond is given by

i=($F-$P_B )/$P_B. (4.2)

Equation (4.2) can be solved for the bond price:

$P_B=$F/(1+i). (4.3)

Given fixed nominal bond payments, equation (4.2) shows that the nominal interest rate and the bond price are inversely related. For example, when the central bank purchases bonds, it increases the demand for them and tends to increase their price, which reduces the interest rate.

Date: 2015-02-16; view: 959