
CATEGORIES:
BiologyChemistryConstructionCultureEcologyEconomyElectronicsFinanceGeographyHistoryInformaticsLawMathematicsMechanicsMedicineOtherPedagogyPhilosophyPhysicsPolicyPsychologySociologySportTourism
TheorMech-2
1. The motion of a particle is described by  . In which moment of time will the velocity of particle be 20 m/s?
. In which moment of time will the velocity of particle be 20 m/s?
· 16
2. Disk of radius R=1.5m rotates with an angular velocity of  , and angular acceleration of
, and angular acceleration of 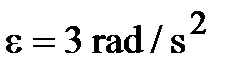 . Determine the acceleration of a point on the rim of the disk.
. Determine the acceleration of a point on the rim of the disk.
· 7.5
3. Which of the points on diagram is the instantaneous centre of zero velocity?
|
|
| L |
| K |
| H |
| N |
| G |
· point N
4. Crank OA of length 1m rotates with angular velocity 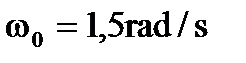 . The length of the connecting rod AB=3m. Determine the velocity of the slide block B at the given instant.
. The length of the connecting rod AB=3m. Determine the velocity of the slide block B at the given instant.

|
| O |
| A |
| B |
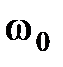
|
· 
5. Give the definition of relative motion of a particle.
· The motion performed by particle with respect to the moving coordinate system
6.
| O |
| R |
| M |

|
| w |
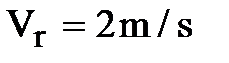 .
.
Determine the absolute velocity of point M (see figure) if the circle rotates with the angular velocity 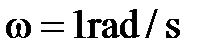 .
.
· 6
7. The motion of a particle is described by the equation  . Find the velocity of particle at time t=5s.
. Find the velocity of particle at time t=5s.
· 23
8. Which of the following equalities expresses uniformly accelerated rotation of a rigid body?
· 
9. Which of the points on diagram is the instantaneous center of zero velocity?
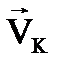
|
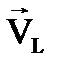
|
| L |
| K |
| H |
| N |
| G |
· point G
10.
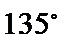
|
| O |
| A |
| B |
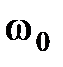
|
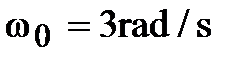 . The length of the connecting rod AB=3m. Determine the velocity of slide block B at the given instant.
. The length of the connecting rod AB=3m. Determine the velocity of slide block B at the given instant.
· 1,8
11.
| z |
| A |
| B |
| O |
| M |

|

|
| w |
 . Particle M moves along the side AB with relative velocity
. Particle M moves along the side AB with relative velocity 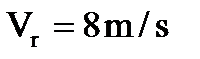 .
.
Determine the absolute velocity of point M (see figure) if the distance AM=2m.
· 
12. The curvilinear motion of a particle is described by equation  (m). Find the radius of curvature at instant when normal acceleration of particle is equal to 5
(m). Find the radius of curvature at instant when normal acceleration of particle is equal to 5 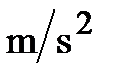 .
.
· 45
13. The tangential acceleration of a point on the rim of disk is equal to 1.5 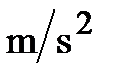 . Determine the angular acceleration of that disk at a given instant, if its radius R=2m.
. Determine the angular acceleration of that disk at a given instant, if its radius R=2m.
· 0.75
14. Crank OA of length 0.5m rotates with angular velocity  . The length of the connecting rod AB=1m. Determine the velocity of the slide block B at the given instant.
. The length of the connecting rod AB=1m. Determine the velocity of the slide block B at the given instant.
| O |
| A |
| B |
|

|
· 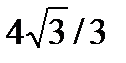
15. Particle M moves along a circle of radius R=2m. At the given instant relative velocity of point M is 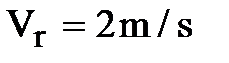 . Determine the absolute velocity of point M (see figure) if circle rotates about axis Oz with the angular velocity
. Determine the absolute velocity of point M (see figure) if circle rotates about axis Oz with the angular velocity  .
.
| z |
| O |
| M |

|
| w |
| R |
· 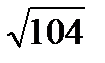
16. A particle moves along a circle of radius R=2m. Equation of particle’s motion is S=10t+5 (m). Find the normal acceleration of particle.
· 50
17. Crank OA of length 0.5m rotates with angular velocity 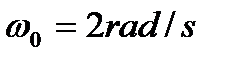 . The length of the connecting rod AB=1m.
. The length of the connecting rod AB=1m.
| O |
| A |
| B |
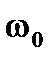
|

|
Determine the velocity of slide block B at the given instant.
· 1
18. Point M moves along a circle of radius R=0.2m. At the given instant relative velocity of point M is  .
.
| O |
| w |
| R |
| M |

|
Determine the absolute velocity of point M (see figure) if circle rotates with angular velocity  .
.
· 2,1
19. The motion of a particle is described by the equations 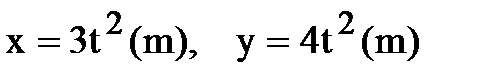 . Find velocity of the particle at time t=2s.
. Find velocity of the particle at time t=2s.
· 20
20. Crank OA of length 0.5m rotates with angular velocity 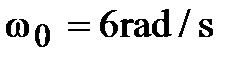 . The length of the connecting rod AB=1m.
. The length of the connecting rod AB=1m.
| O |
| A |
| B |
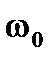
|

|
Determine the angular velocity of rod AB at the given instant.
· 3
21. The normal acceleration of a point on the rim of disk is equal to 2 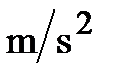 . Determine the angular velocity of that disk at given instant, if its radius R=0.5m.
. Determine the angular velocity of that disk at given instant, if its radius R=0.5m.
· 2
22. Motion of a particle is described by the equations 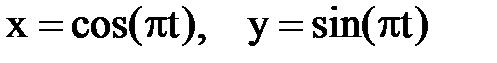 . Find the acceleration of particle.
. Find the acceleration of particle.
· 
23. Indicate the formula defining the velocity vector of point B in plane motion.
· 
24.
| B |
| A |

|
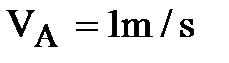 .
.
· 
25. Triangle ABC rotates about axis AC with angular velocity  . Point M moves along the side BC with relative velocity
. Point M moves along the side BC with relative velocity  .
.
| A |
| B |
| M |

|
| w |
| С |
| a |
Determine the Coriolis acceleration if 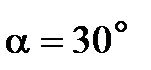 .
.
· 12
26. The equations of motion of a particle: 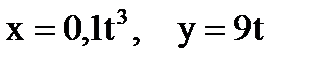 . Find velocity of the particle at instant t=20s.
. Find velocity of the particle at instant t=20s.
· 15
27. The equation of rotation of a rigid body given by 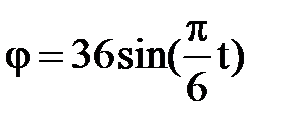 . Determine the angular acceleration at time t=1s.
. Determine the angular acceleration at time t=1s.
· – 0,5 p2
28. What is the velocity of point B of the wheel rolling without slipping,
| B |
| A |

|
if the centre A has at a given instant the velocity 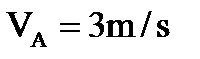 .
.
· 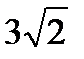
29.
| z |
| O |
| M |

|
| w |
| A |
| B |
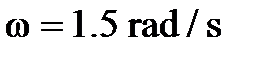 . Point M moves along the rod AB with the relative velocity
. Point M moves along the rod AB with the relative velocity 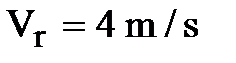 .
.
What is the Coriolis acceleration of point M?
· 12
30. The equation of rotation is 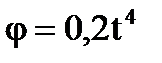 . Find the angular acceleration at time t=6s.
. Find the angular acceleration at time t=6s.
· 86,4
31. Point A of the step-wheel has at a given instant velocity 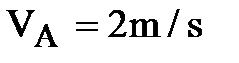 . Radii are:
. Radii are:  .
.
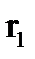
|
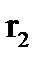
|
| A |
| B |
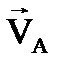
|
What is the velocity of point B?
· 2
32. Triangle ABC rotates about axis Oz with angular velocity  . Point M moves along the side AC with relative velocity
. Point M moves along the side AC with relative velocity 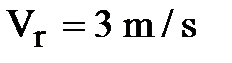 .
.
| z |
| A |
| B |
| O |
| M |

|

|
| w |
| С |
What is the Coriolis acceleration of point M?
· 3
33. The tangential acceleration of a point on the rim of the disk is 1.5 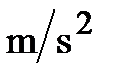 . Determine the angular acceleration of the disk, if its radius R=3/2m.
. Determine the angular acceleration of the disk, if its radius R=3/2m.
· 1
34. A particle is moving along the curvilinear trajectory. Its equation of motion given by  m. Determine the radius of curvature at time when normal acceleration of the particle
m. Determine the radius of curvature at time when normal acceleration of the particle  =20
=20  .
.
· 5
35. Rod АВ of length 1m is moving as indicated between the wall and floor. At the indicated position point A has velocity 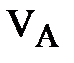 =2 m/s. What is the angular velocity of AB?
=2 m/s. What is the angular velocity of AB?
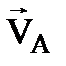
|
| А |
| В |

|
· 4
36.
| О |
| А |
| М |
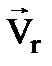
|

|
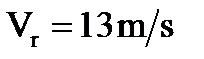 , while OA itself rotates in the plane around point O with the angular velocity
, while OA itself rotates in the plane around point O with the angular velocity 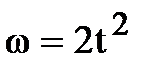 rad/s.
rad/s.
Determine the Coriolis acceleration at time t=2s.
· 208
37. Normal acceleration of point M of the disc rotating about fixed axis is equal to 6.4m/s2. What is the angular velocity 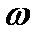 of the disc if its radius R=0.9m.
of the disc if its radius R=0.9m.
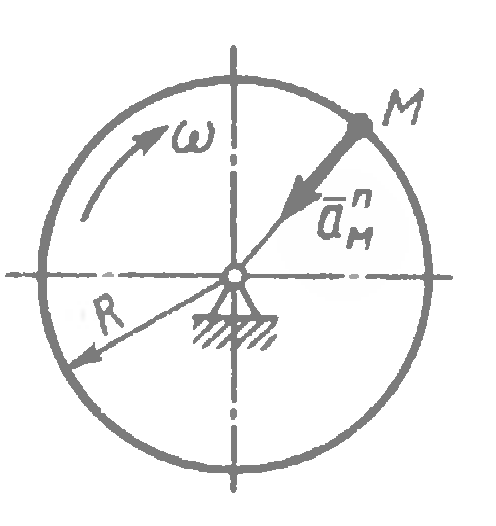
· 8/3
38. Velocity of a car is 90km/h. Find the distance (in meter) when car stops if it decelerates with the constant acceleration 5m/s2.
· 62.5
39. Acceleration of the rectilinear motion of a point is 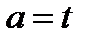 . Initial velocity of the point is
. Initial velocity of the point is 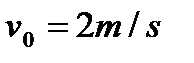 . Find velocity of the point at time t=10s.
. Find velocity of the point at time t=10s.
· 52
40. A point is in rectilinear motion with the acceleration a=0.2t. Find the time t at which the velocity of the point will be equal 2m/s. Initial velocity v0=0.
· 4.47
41. An aircraft flies by the circular trajectory of the radius r=10km. Find the velocity of the aircraft in km/h if its normal acceleration 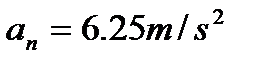 .
.
· 900
42. Point moves along the circle of radius R=5m according to the equation s=0.5t2 . Find coordinate s of the point at instant when normal acceleration an=5m/s2.
· 12.5
43. Find normal acceleration of a point at instant when its acceleration a=1.8m/s2, and angle between acceleration vector and velocity vector is equal to 300.
· 0.9
44. Angular velocity of a body is given by 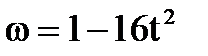 . What time does the body need to stop?
. What time does the body need to stop?
· 0.25
45. A body rotates about fixed axis by  . Find tangential acceleration of the point of the body at time t=4s if radius of rotation of the point R=0.25m.
. Find tangential acceleration of the point of the body at time t=4s if radius of rotation of the point R=0.25m.
· 0.5
46. Rod AB of length 80cm moves in the plane. At the given instant velocities of the points A and B are equal vA=0.2m/s and vB=0.6m/s respectively. Find the angular velocity of the rod AB.
| A |
| B |
| VA |
| VB |
· 0.4
47. Triangle is rotating about side AB with the angular velocity ω. Point M moves along the side of the triangle with the relative velocity  . Find the relative acceleration of point M at time t=6s.
. Find the relative acceleration of point M at time t=6s.
· 60
48. Rod AB performs plane motion. In the given position of the rod velocities of points A and B are 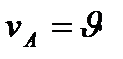 ,
, 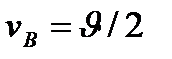 . What is the angle between velocity-vector
. What is the angle between velocity-vector 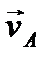 and the line AB?
and the line AB?
· 600
49. Rod AB performs plane motion. АВ=2m, angular velocity of the rod 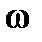 =1rad/s, angular acceleration
=1rad/s, angular acceleration  =0, acceleration of point А is
=0, acceleration of point А is 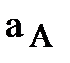 =2m/s2.
=2m/s2.

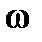
Find the acceleration of point B.
· 4
50. 

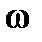
 A body (see figure) performs plane motion. АВ=0.5m, angular velocity
A body (see figure) performs plane motion. АВ=0.5m, angular velocity 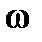 =1rad/s, angular acceleration
=1rad/s, angular acceleration  =0. Acceleration of point А at the given instant is
=0. Acceleration of point А at the given instant is 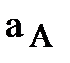 =3m/s2.
=3m/s2.
Determine the acceleration of point B.
· 2.5
51. Rod AB is in plane motion. АВ=40сm, 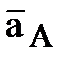 =2m/
=2m/ 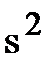 ,
,  =6 m/
=6 m/ 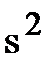 .
.

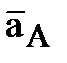


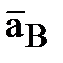
Find the angular acceleration of the rod AB.
· 10
52. A body performs plane motion. АВ=1m, 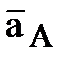 =1m/
=1m/ 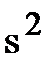 ,
,  =8 m/
=8 m/ 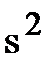 .
.

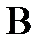
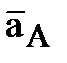

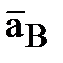
Find the angular velocity of the body.
· None of these
53. Rod AB performs plane motion. АВ=2m, angular velocity of the rod is 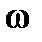 =1rad/s, angular acceleration
=1rad/s, angular acceleration  =0, acceleration of point А is
=0, acceleration of point А is 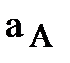 =0.6m/s2.
=0.6m/s2.

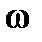

Determine the acceleration of point B.
· 1.6
54. A body (see diagram) is in plane motion. АВ=1m, 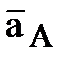 =2m/
=2m/ 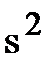 ,
,  = 10m/
= 10m/ 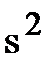 .
.

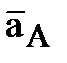

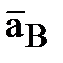

Find the angular velocity of the body.
· 6.7
55.
| O |
| R |
| M |

|
| w |
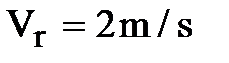 .
.
Determine the absolute velocity of point M (see figure) if the circle rotates with the angular velocity 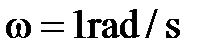 .
.
· 2.4
56.
| z |
| A |
| B |
| O |
| M |

|

|
| w |
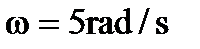 . Particle M moves along the side AB with relative velocity
. Particle M moves along the side AB with relative velocity  .
.
Determine the absolute velocity of point M (see figure) if the distance AM=2m.
· 
57. Crank OA of length 0.5m rotates with angular velocity 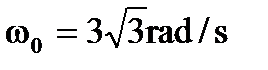 . The length of the connecting rod AB=1m. Determine the angular velocity of the rod AB at the given instant.
. The length of the connecting rod AB=1m. Determine the angular velocity of the rod AB at the given instant.
| O |
| A |
| B |
|

|
· 3
58. Law of motion of a point is given by 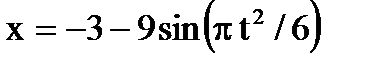 ,
,  . What trajectory is described by the point?
. What trajectory is described by the point?
· Circle
59. Velocity of a load 1 is V. Find angular velocity of the pulley-3.
· V/3r
60. Find angular velocity of a rod AB if 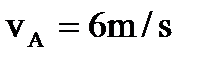 .
.
· 6
61. Disc of radius R=10 m/s rotates about axis Ox with the law 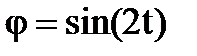 rad. Find velocity of a point on the rim of the disc at time t = π sec.
rad. Find velocity of a point on the rim of the disc at time t = π sec.
· 20
62. A law of motion of a particle is given by s=5 + 2.5t2 . Find velocity of the particle at time t=36s.
· 180
63. Projections of the velocity vector of a point on the coordinate axes are given:  ,
,  ,
, 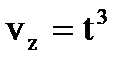 . Find magnitude of the acceleration vector of the point at time t=1sec.
. Find magnitude of the acceleration vector of the point at time t=1sec.
· 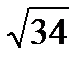
64. A square plate rotates about horizontal axis according to a law 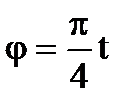 (rad). Point M is moving along a diagonal by a law
(rad). Point M is moving along a diagonal by a law  (m).
(m).
Find Coriolis acceleration of the point M.
· 
Date: 2015-01-29; view: 3831
| <== previous page | | | next page ==> |
| TheorMech-3 | | | The Parliament of Australia |